Exemplar Solutions NCERT Solutions

Integrals-Short Answer Type:Q 1-5

Verify the following:

Question (1) `int(2x-1)/(2x+3)dx` `=-log|(2x+3)^2|+C`

Solution:

Let, `I=int(2x-1)/(2x+3)dx`

`=int(2x+3-4)/(2x+3)dx`

`=int((2x+3)/(2x+3)-4/(2x+3))dx`

`=int (1-4/(2x+3))dx`

`=int[1-4/(2(x+3/2))]dx`

`=int[1-2/(x+3/2)]dx`

`=x-2log|x+3/2|+c`

`=x-2log|(2x+3)/2|+c`

[∵ `log(m/n)=logm -logn`]

`:. x-2log|(2x+3)/2|+c` `=x-2log|2x+3|+2log|2|+c`

`=x-log|(2x+3)^2|+log4+c`

`=x-log|(2x+3)^2|+c`

Hence, proved

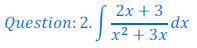
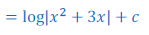
Solution:

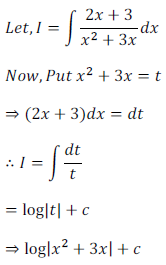
Hence, Proved.

Evaluate the following:

12-math-home

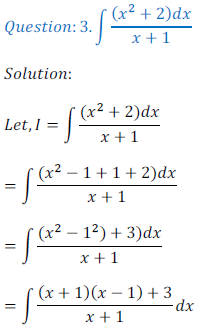
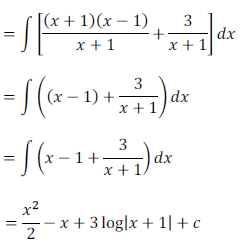
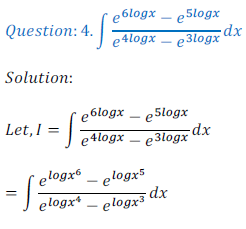
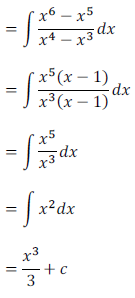
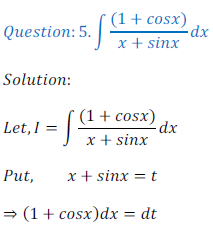
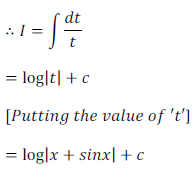
Reference: