Electricity - Class 10th Science

NCERT Exercise Solution-1

Question: 7. The values of current I flowing in a given resistor for the corresponding values of potential difference V across the resistor are given below –

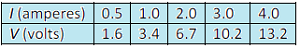
Plot a graph between V and I and calculate the resistance of that resistor.

Answer:

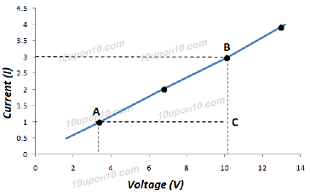
We know that, slope of the graph will give the value of resistance.

Now, let take two points A and B and draw AC parallel to y-axis and BC parallel to x-axis

BC = 10.2 V – 3.4 V = 6.8 V

AC = 3 –1 = 2 ampere

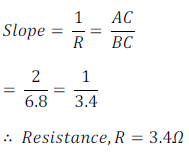
Question: 8. When a 12 V battery is connected across an unknown resistor, there is a current of 2.5 mA in the circuit. Find the value of the resistance of the resistor.

Answer:

Given,

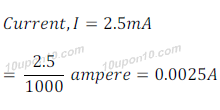
Potential difference, V = 12V,

Thus, resistance, R =?

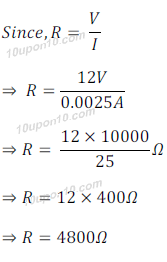
Thus, resistance = 4800Ω Answer

Question: 9. A battery of 9 V is connected in series with resistors of 0.2 Ω, 0.3 Ω, 0.4 Ω , 0.5 Ω and 12 Ω, respectively. How much current would flow through the 12 Ω resistor?

Answer:

Since, resistors are connected in series, thus total resistance of the circuit will be equal to sum of all the resistors.

Let, total effective resistance of the circuit = R

Therefore, R= 0.2 Ω + 0.3 Ω + 0.4 Ω + 0.5 Ω + 12 Ω

Or, R = 13.4 Ω

Now, electric current drawn through the circuit,

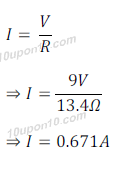
Since, electric current does not divided in series circuit, thus, electric current flows through the resistors of 12Ω = 0.671A

Question: 10. How many 176 Ω resistors (in parallel) are required to carry 5 A on a 220 V line?

Answer:

Given, I = 5 A and V = 200V

Let, total equivalent resistance after connecting x resisters in parallel = R₁

Therefore, R₁ = 220V / 5A

Let, total number required resistors of 176 Ω = x

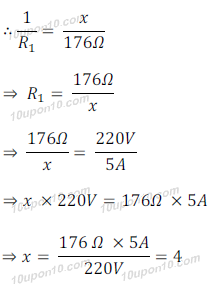
Thus, 4 resistors of 176Ω are required.

Hence, Answer = 4

Question: 11. Show how you would connect three resistors, each of resistance 6 Ω, so that the combination has a resistance of (i) 9 Ω, (ii) 4 Ω.

Answer:

Given resistance can be connected in following possible ways

(a) When all the three is connected in series

Therefore, total effective resistance = 6Ω x 3 = 18 Ω

(b) When all the three is connected in parallel

Let, total effective resistance = R

(c) By connecting two resistance in series and one in parallel

When two resistance are connected in series, then equivalent resistance = 6Ω+6Ω=12Ω

And third is connected in parallel

Let the effective resistance = R¹

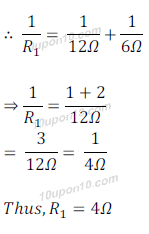
(d) When two are connected in parallel and one is connected in series

Let the total effective resistance when two are connected in parallel = R₂

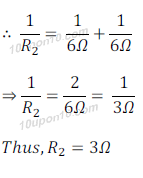
Now, third is connected in series with this R₂

Therefore, total effective resistance = 3Ω + 6Ω = 9Ω

(i) When two are connected in parallel and one in series, therefore, total resistance = 9Ω

(ii) When two are connected in series and one in parallel, therefore, total resistance = 4Ω

• ❮ Previous
• Next ❯